"Experimental and theoretical study on multicomponent diffusion gases under rarefied conditions"
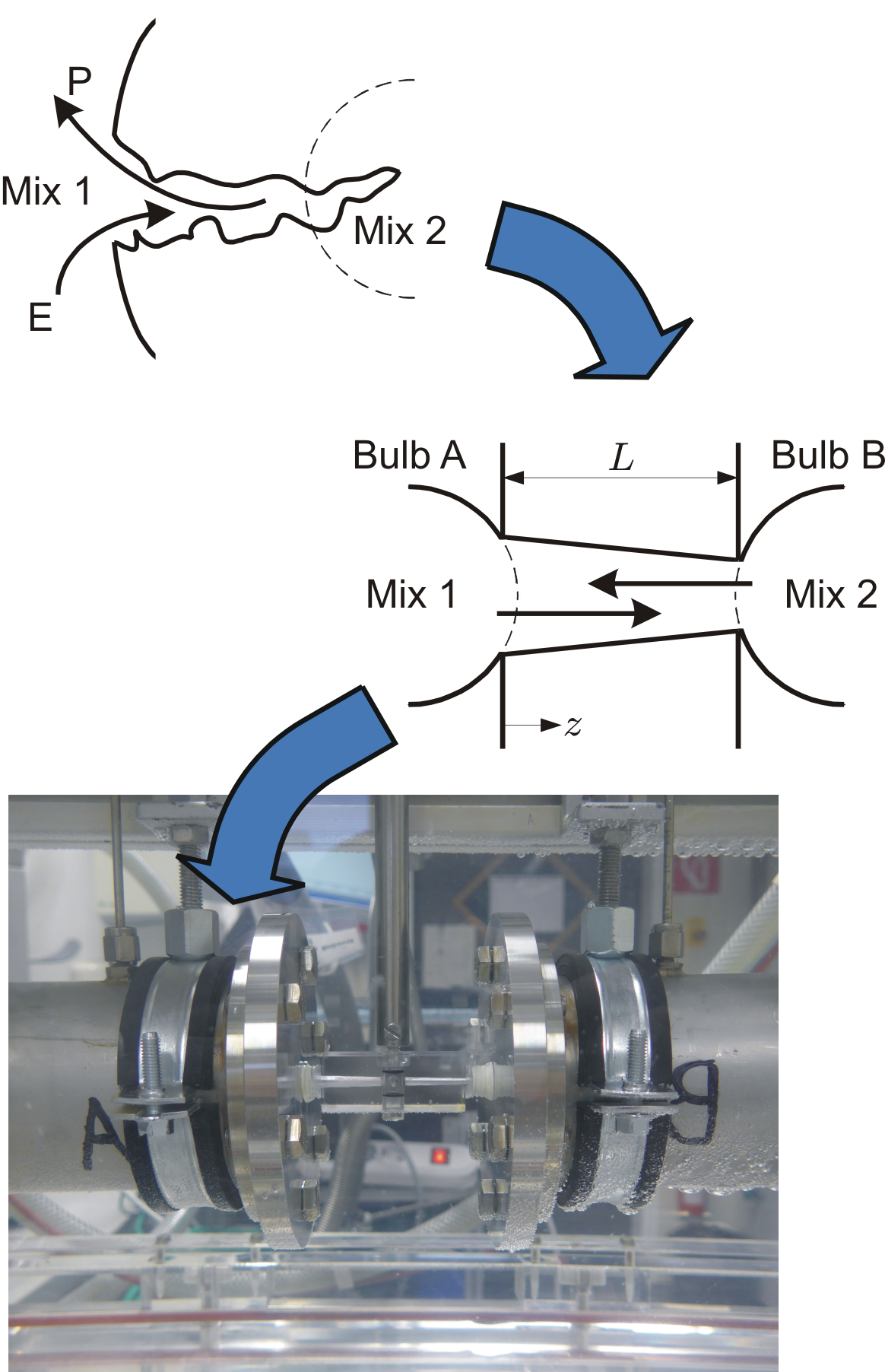
Multicomponent diffusion of gases in microscale and nanoscale confined geometries is determining the integral behaviour and efficiency of many natural and technical processes. In such small systems the gas is in a state referred to as rarefied where diffusion is the dominating, and hence limiting, transport mechanism. Considerable enhancement on the macroscale in, e.g., membrane gas separation, heterogeneous catalysis, and microelectromechanical systems is only possible when understanding the dependence of gaseous rarefaction on multicomponent diffusion fundamentally. The Maxwell-Stefan equations (MSE) yield reasonable results for bulk multicomponent diffusion but fail for the description of transport under rarefied conditions. Runstedtler’s approach from 2006 superimposes bulk diffusion and Knudsen diffusion to the MSE. However, in this approach surface diffusion is disregarded which, according to my own work, under higher orders of rarefaction is the dominating diffusion mechanism. The nowadays accepted Binary Friction Model (BFM) contains a correction factor that accounts for the transition between the continuum regime and the free molecular regime. This indicates that the BFM does not fully explain the mechanisms yet, and potential for improvement is given. Furthermore, in literature all experimental and numerical analysis on multicomponent diffusion confine on uniform ducts as object of examination. This is wondrous since “real” pores are mostly tapered and diffusive flux strongly depends on the duct’s cross-sectional area as it was shown in an own earlier work. This project aims for a new, experimentally validated model that allows for the prediction of multicomponent diffusion in uniform and tapered ducts over a wide range of gaseous rarefaction.
Contact: Thöming, Veltzke